P Or Q Then R

Truth Tables, Tautologies, and Logical Equivalences

Mathematicians normally use a two-valued logic: Every argument is either True or False. This is chosen the Police force of the Excluded Centre.

A statement in sentential logic is built from simple statements using the logical connectives $\lnot$ , $\land$ , $\lor$ , $\ifthen$ , and $\iff$ . The truth or falsity of a statement built with these connective depends on the truth or falsity of its components.

For example, the compound statement $P \ifthen (Q \lor \lnot R)$ is built using the logical connectives $\ifthen$ , $\lor$ , and $\lnot$ . The truth or falsity of $P \ifthen (Q \lor \lnot R)$ depends on the truth or falsity of P, Q, and R.

A truth table shows how the truth or falsity of a compound statement depends on the truth or falsity of the simple statements from which it'south synthetic. And so nosotros'll get-go by looking at truth tables for the v logical connectives.

Here's the table for negation:

$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & \cr & P & & $\lnot P$ & \cr height2pt & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & \cr & T & & F & \cr height2pt & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & \cr & F & & T & \cr height2pt & \omit & & \omit & \cr \noalign{\hrule} }}$

This table is piece of cake to understand. If P is truthful, its negation $\lnot P$ is fake. If P is false, and so $\lnot P$ is truthful.

$P \land Q$ should be true when both P and Q are true, and faux otherwise:

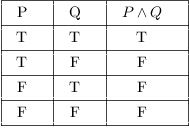
$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & P & & Q & & $P \land Q$ & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & T & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

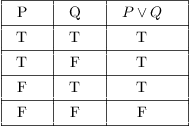
$P \lor Q$ is true if either P is true or Q is true (or both --- call back that we're using "or" in the inclusive sense). It's just false if both P and Q are false.

$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & P & & Q & & $P \lor Q$ & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & F & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

Hither's the table for logical implication:

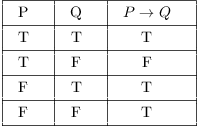
$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & P & & Q & & $P \ifthen Q$ & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & F & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

To understand why this tabular array is the way it is, consider the following example:

"If you get an A, so I'll requite yous a dollar."

The statement will exist true if I keep my promise and false if I don't.

Suppose it's true that you lot go an A and information technology'south truthful that I give you a dollar. Since I kept my promise, the implication is truthful. This corresponds to the beginning line in the table.

Suppose it'southward truthful that you go an A only it'south imitation that I requite you a dollar. Since I didn't keep my promise, the implication is simulated. This corresponds to the second line in the table.

What if it's simulated that you get an A? Whether or not I give you lot a dollar, I oasis't cleaved my promise. Thus, the implication tin can't be false, so (since this is a two-valued logic) it must be true. This explains the last two lines of the tabular array.

$P \iff Q$ means that P and Q are equivalent. And so the double implication is truthful if P and Q are both true or if P and Q are both faux; otherwise, the double implication is simulated.

$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & P & & Q & & $P \iff Q$ & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & T & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & F & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

Yous should call up --- or be able to construct --- the truth tables for the logical connectives. You'll use these tables to construct tables for more than complicated sentences. It's easier to demonstrate what to do than to depict it in words, and then you'll see the procedure worked out in the examples.

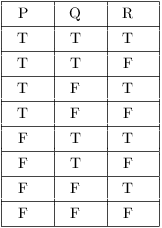
Remark. (a) When y'all're constructing a truth tabular array, you have to consider all possible assignments of True (T) and Simulated (F) to the component statements. For case, suppose the component statements are P, Q, and R. Each of these statements can be either truthful or simulated, so there are $2^3 = 8$ possibilities.

When you're list the possibilities, y'all should assign truth values to the component statements in a systematic way to avert duplication or omission. The easiest approach is to use lexicographic ordering. Thus, for a chemical compound statement with iii components P, Q, and R, I would list the possibilities this mode:

$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & P & & Q & & R & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & T & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & F & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & T & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & T & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & T & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & F & & T & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & \cr & F & & F & & F & \cr height2pt & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

(b) At that place are dissimilar means of setting upward truth tables. You lot can, for case, write the truth values "under" the logical connectives of the compound statement, gradually building up to the column for the "primary" connective.

I'll write things out the long way, by amalgam columns for each "piece" of the chemical compound statement and gradually edifice up to the compound statement. Whatever style is fine as long as you prove plenty piece of work to justify your results.

Example. Construct a truth tabular array for the formula $\lnot P \land (P \ifthen Q)$ .

First, I list all the alternatives for P and Q.

Next, in the third cavalcade, I listing the values of $\lnot P$ based on the values of P. I use the truth tabular array for negation: When P is true $\lnot P$ is false, and when P is false, $\lnot P$ is truthful.

In the 4th column, I list the values for $P \ifthen Q$ . Bank check for yourself that it is only fake ("F") if P is truthful ("T") and Q is fake ("F").

The fifth cavalcade gives the values for my chemical compound expression $\lnot P \land (P \ifthen Q)$ . It is an "and" of $\lnot P$ (the third column) and $P \ifthen Q$ (the 4th cavalcade). An "and" is true only if both parts of the "and" are true; otherwise, it is imitation. So I look at the 3rd and fourth columns; if both are true ("T"), I put T in the fifth column, otherwise I put F.

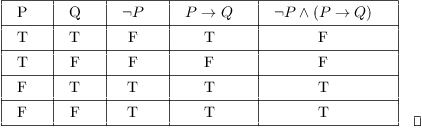
$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & P & & Q & & $\lnot P$ & & $P \ifthen Q$ & & $\lnot P \land (P \ifthen Q)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & T & & F & & T & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & F & & F & & F & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & T & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & F & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}\quad\halmos$

A tautology is a formula which is "e'er true" --- that is, information technology is true for every assignment of truth values to its unproblematic components. Y'all tin think of a tautology as a rule of logic.

The opposite of a tautology is a contradiction, a formula which is "ever false". In other words, a contradiction is false for every assignment of truth values to its simple components.

Example. Prove that $(P \ifthen Q) \lor (Q \ifthen P)$ is a tautology.

I construct the truth table for $(P \ifthen Q) \lor (Q \ifthen P)$ and show that the formula is always truthful.

$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & P & & Q & & $P \ifthen Q$ & & $Q \ifthen P$ & & $(P \ifthen Q) \lor (Q \ifthen P)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & T & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & F & & F & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & T & & T & & F & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & F & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

The concluding column contains simply T's. Therefore, the formula is a tautology.

Instance. Construct a truth table for $(P \ifthen Q) \land (Q \ifthen R)$ .

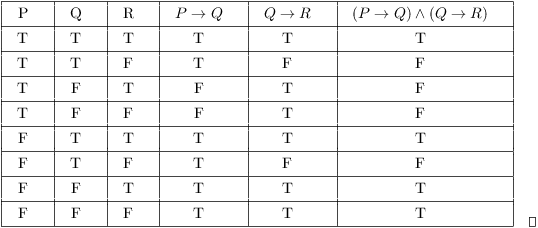
$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & P & & Q & & R & & $P \ifthen Q$ & & $Q \ifthen R$ & & $(P \ifthen Q) \land (Q \ifthen R)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & T & & T & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & T & & F & & T & & F & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & F & & T & & F & & T & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & F & & F & & F & & T & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & T & & T & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & T & & F & & T & & F & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & F & & T & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & F & & F & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}\quad\halmos$

Yous tin encounter that amalgam truth tables for statements with lots of connectives or lots of simple statements is pretty tedious and error-prone. While at that place might be some applications of this (due east.thou. to digital circuits), at some indicate the all-time thing would be to write a programme to construct truth tables (and this has surely been washed).

The point here is to sympathize how the truth value of a complex statement depends on the truth values of its simple statements and its logical connectives. In most piece of work, mathematicians don't ordinarily utilize statements which are very complicated from a logical bespeak of view.

Example. (a) Suppose that P is false and $P \lor \lnot Q$ is true. Tell whether Q is true, false, or its truth value can't be determined.

(b) Suppose that $(P \land \lnot Q) \ifthen R$ is fake. Tell whether Q is truthful, false, or its truth value tin't be determined.

(a) Since $P \lor \lnot Q$ is true, either P is true or $\lnot Q$ is truthful. Since P is false, $\lnot Q$ must be true. Hence, Q must be false.

(b) An if-then statement is fake when the "if" part is truthful and the "then" function is false. Since $(P \land \lnot Q) \ifthen R$ is false, $P \land \lnot Q$ is true. An "and" statement is true simply when both parts are true. In particular, $\lnot Q$ must be true, and so Q is fake.

Case. Suppose

" $x > y$ " is truthful.

" $\displaystyle \int f(x)\,dx = g(x) + C$ " is false.

"Calvin Butterball has purple socks" is true.

Make up one's mind the truth value of the argument

$(x > y \ifthen \int f(x)\,dx = g(x) + C) \ifthen\,\lnot (\hbox{Calvin Butterball has purple socks})$

For simplicity, allow

P = " $x > y$ ".

Q = " $\displaystyle \int f(x)\,dx = g(x) + C$ ".

R = "Calvin Pudgy has purple socks".

I want to make up one's mind the truth value of $(P \ifthen Q) \ifthen\,\lnot R$ . Since I was given specific truth values for P, Q, and R, I gear up a truth table with a single row using the given values for P, Q, and R:

Therefore, the statement is truthful.

Example. Determine the truth value of the statement

$(10 > 42) \ifthen \hbox{``Ichabod Xerxes eats chocolate cupcakes''}$

The statement " $10 > 42$ " is faux. Y'all tin't tell whether the statement "Ichabod Xerxes eats chocolate cupcakes" is true or false --- just it doesn't thing. If the "if" part of an "if-and so" argument is false, then the "if-so" statement is true. (Check the truth table for $P \ifthen Q$ if you're not certain almost this!) So the given statement must be true.

Two statements 10 and Y are logically equivalent if $X \iff Y$ is a tautology. Another way to say this is: For each assignment of truth values to the simple statements which make upwardly X and Y, the statements X and Y have identical truth values.

From a practical point of view, you can supplant a statement in a proof by any logically equivalent argument.

To test whether X and Y are logically equivalent, yous could set upwardly a truth tabular array to test whether $X \iff Y$ is a tautology --- that is, whether $X \iff Y$ "has all T's in its column". Yet, information technology's easier to prepare up a tabular array containing Ten and Y then cheque whether the columns for X and for Y are the same.

Case. Prove that $P \ifthen Q$ and $\lnot P \lor Q$ are logically equivalent.

$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & P & & Q & & $P \ifthen Q$ & & $\lnot P$ & & $\lnot P \lor Q$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & T & & T & & F & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & T & & F & & F & & F & & F & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & T & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr & F & & F & & T & & T & & T & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }}$

Since the columns for $P \ifthen Q$ and $\lnot P \lor Q$ are identical, the 2 statements are logically equivalent. This tautology is chosen Conditional Disjunction. You tin use this equivalence to supersede a conditional by a disjunction.

There are an infinite number of tautologies and logical equivalences; I've listed a few below; a more than extensive list is given at the end of this department.

$\matrix{ \hbox{Double negation} & \lnot(\lnot P) \iff P \cr \hbox{DeMorgan's Law} & \lnot(P \lor Q) \iff (\lnot P \land \lnot Q) \cr \hbox{DeMorgan's Law} & \lnot(P \land Q) \iff (\lnot P \lor \lnot Q) \cr \hbox{Contrapositive} & (P \ifthen Q) \iff (\lnot Q \ifthen\,\lnot P) \cr \hbox{Modus ponens} & [P \land (P \ifthen Q)] \ifthen Q \cr \hbox{Modus tollens} & [\lnot Q \land (P \ifthen Q)] \ifthen\,\lnot P \cr}$

When a tautology has the form of a biconditional, the two statements which make upwards the biconditional are logically equivalent. Hence, you tin supervene upon ane side with the other without changing the logical meaning.

Yous volition often need to negate a mathematical statement. To see how to do this, we'll begin by showing how to negate symbolic statements.

Example. Write downward the negation of the following statements, simplifying so that only simple statements are negated.

(a) $(P \lor \lnot Q)$

(b) $(P \land Q) \ifthen R$

(a) I negate the given statement, and so simplify using logical equivalences. I've given the names of the logical equivalences on the correct so yous can see which ones I used.

$\matrix{\lnot(P \lor \lnot Q) & \iff & \lnot P \land \lnot\lnot Q & \hbox{DeMorgan's law} \cr & \iff & \lnot P \land Q & \hbox{Double negation} \cr}\quad\halmos$

(b)

$\matrix{\lnot[(P \land Q) \ifthen R] & \iff & \lnot[\lnot(P \land Q) \lor R] & \hbox{Conditional Disjunction} \cr & \iff & \lnot\lnot(P \land Q) \land \lnot R & \hbox{DeMorgan's law} \cr & \iff & (P \land Q) \land \lnot R & \hbox{Double negation} \cr}$

I showed that $(A \ifthen B)$ and $(\lnot A \lor B)$ are logically equivalent in an before example.

In the following examples, we'll negate statements written in words. This is more typical of what you lot'll need to do in mathematics. The thought is to convert the word-statement to a symbolic statement, then use logical equivalences as nosotros did in the last example.

Instance. Use DeMorgan's Law to write the negation of the following statement, simplifying and then that just elementary statements are negated:

"Calvin is not dwelling or Bonzo is at the movies."

Let C exist the argument "Calvin is home" and let B be the statement "Bonzo is at the moves". The given statement is $\lnot C \lor B$ . I'm supposed to negate the statement, then simplify:

$\matrix{\lnot(\lnot C \lor B) & \iff & \lnot\lnot C \land \lnot B & \hbox{DeMorgan's Law} \cr & \iff & C \land \lnot B & \hbox{Double negation} \cr}$

The consequence is "Calvin is home and Bonzo is not at the movies".

Instance. Use DeMorgan's Law to write the negation of the post-obit argument, simplifying so that but uncomplicated statements are negated:

"If Phoebe buys a pizza, so Calvin buys popcorn."

Permit P be the statement "Phoebe buys a pizza" and let C exist the statement "Calvin buys popcorn". The given statement is $P \ifthen C$ . To simplify the negation, I'll use the Provisional Disjunction tautology which says

$(P \ifthen Q) \iff (\lnot P \lor Q)$

That is, I can supervene upon $P \ifthen Q$ with $\lnot P \lor Q$ (or vice versa).

Here, then, is the negation and simplification:

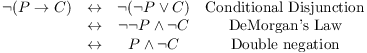
$\matrix{\lnot(P \ifthen C) & \iff & \lnot(\lnot P \lor C) & \hbox{Conditional Disjunction} \cr & \iff & \lnot\lnot P \land \lnot C & \hbox{DeMorgan's Law} \cr & \iff & P \land \lnot C & \hbox{Double negation} \cr}$

The consequence is "Phoebe buys the pizza and Calvin doesn't buy popcorn".

Side by side, we'll employ our work on truth tables and negating statements to issues involving constructing the converse, inverse, and contrapositive of an "if-then" statement.

Instance. Replace the following statement with its contrapositive:

"If 10 and y are rational, then $x + y$ is rational."

Past the contrapositive equivalence, this statement is the same as "If $x + y$ is not rational, then information technology is not the case that both x and y are rational".

This reply is correct as information technology stands, but we can express it in a slightly better way which removes some of the explicit negations. Most people find a positive statement easier to encompass than a negative statement.

By definition, a existent number is irrational if it is not rational. And so I could replace the "if" part of the contrapositive with " $x + y$ is irrational".

The "then" office of the contrapositive is the negation of an "and" statement. You could restate it as "It's not the case that both x is rational and y is rational". (The word "both" ensures that the negation applies to the whole "and" statement, not just to "x is rational".)

By DeMorgan's Police, this is equivalent to: "x is non rational or y is not rational". Alternatively, I could say: "ten is irrational or y is irrational".

Putting everything together, I could express the contrapositive as: "If $x + y$ is irrational, then either x is irrational or y is irrational".

(As usual, I added the word "either" to go far clear that the "and then" part is the whole "or" argument.)

Example. Bear witness that the changed and the converse of a provisional are logically equivalent.

Let $P \ifthen Q$ be the conditional. The changed is $\lnot P \ifthen\,\lnot Q$ . The converse is $Q \ifthen P$ .

I could show that the inverse and converse are equivalent past constructing a truth table for $(\lnot P \ifthen\,\lnot Q) \iff (Q \ifthen P)$ . I'll use some known tautologies instead.

Start with $\lnot P \ifthen\,\lnot Q$ :

$\matrix{\lnot P \ifthen\,\lnot Q & \iff & \lnot\lnot Q \ifthen \lnot\lnot P & \hbox{Contrapositive} \cr & \iff & Q \ifthen P & \hbox{Double negation} \cr}$

Remember that I can replace a statement with 1 that is logically equivalent. For example, in the last step I replaced $\lnot\lnot Q$ with Q, because the ii statements are equivalent by Double negation.

Example. Suppose 10 is a real number. Consider the argument

"If $x^2 = 4$ , so $x = 2$ ."

Construct the antipodal, the inverse, and the contrapositive. Determine the truth or falsity of the 4 statements --- the original statement, the converse, the inverse, and the contrapositive --- using your knowledge of algebra.

The converse is "If $x = 2$ , then $x^2 = 4$ ".

The changed is "If $x^2 \ne 4$ , then $x \ne 2$ ".

The contrapositive is "If $x \ne 2$ , then $x^2 \ne 4$ ".

The original statement is false: $(-2)^2 = 4$ , merely $-2 \ne 2$ . Since the original statement is eqiuivalent to the contrapositive, the contrapositive must exist false as well.

The antipodal is true. The inverse is logically equivalent to the converse, so the inverse is true also.

\newpage

\centerline{\bigssbold List of Tautologies}

$\matrix{ 1. \hfill & P \lor \lnot P \hfill & \hbox{Law of the excluded middle} \hfill \cr 2. \hfill & \lnot(P \land \lnot P) \hfill & \hbox{Contradiction} \hfill \cr 3. \hfill & [(P \ifthen Q) \land \lnot Q] \ifthen \lnot P \hfill & \hbox{ Modus tollens} \hfill \cr 4. \hfill & \lnot\lnot P \iff P \hfill & \hbox{Double negation} \hfill \cr 5. \hfill & [(P \ifthen Q) \land (Q \ifthen R)] \ifthen (P \ifthen R) \hfill & \hbox{Law of the syllogism} \hfill \cr 6. \hfill & (P \land Q) \ifthen P \hfill & \hbox{Decomposing a conjunction} \hfill \cr & (P \land Q) \ifthen Q \hfill & \hbox{Decomposing a conjunction} \hfill \cr 7. \hfill & P \ifthen (P \lor Q) \hfill & \hbox{Constructing a disjunction} \hfill \cr & Q \ifthen (P \lor Q) \hfill & \hbox{Constructing a disjunction} \hfill \cr 8. \hfill & (P \iff Q) \iff [(P \ifthen Q) \land (Q \ifthen P)] \hfill & \hbox{Definition of the biconditional} \hfill \cr 9. \hfill & (P \land Q) \iff (Q \land P) \hfill & \hbox{Commutative law for $\land$} \hfill \cr 10. \hfill & (P \lor Q) \iff (Q \lor P) \hfill & \hbox{Commutative law for $\lor$} \hfill \cr 11. \hfill & [(P \land Q) \land R] \iff [P \land (Q \land R)] \hfill & \hbox{Associative law for $\land$} \hfill \cr 12. \hfill & [(P \lor Q) \lor R] \iff [P \lor (Q \lor R)] \hfill & \hbox{Associative law for $\lor$} \hfill \cr 13. \hfill & \lnot(P \lor Q) \iff (\lnot P \land \lnot Q) \hfill & \hbox{DeMorgan's law} \hfill \cr 14. \hfill & \lnot(P \land Q) \iff (\lnot P \lor \lnot Q) \hfill & \hbox{DeMorgan's law} \hfill \cr 15. \hfill & [P \land (Q \lor R)] \iff [(P \land Q) \lor (P \land R)] \hfill & \hbox{Distributivity} \hfill \cr 16. \hfill & [P \lor (Q \land R)] \iff [(P \lor Q) \land (P \lor R)] \hfill & \hbox{Distributivity} \hfill \cr 17. \hfill & (P \ifthen Q) \iff (\lnot Q \ifthen \lnot P) \hfill & \hbox{Contrapositive} \hfill \cr 18. \hfill & (P \ifthen Q) \iff (\lnot P \lor Q) \hfill & \hbox{Conditional disjunction} \hfill \cr 19. \hfill & [(P \lor Q) \land \lnot P] \ifthen Q \hfill & \hbox{Disjunctive syllogism} \hfill \cr 20. \hfill & (P \lor P) \iff P \hfill & \hbox{Simplification} \hfill \cr 21. \hfill & (P \land P) \iff P \hfill & \hbox{Simplification} \hfill \cr}$